Fractals in the Body
Not only are fractals in the world all around us - they are even INSIDE us!
In fact, many of our internal organs and structures display fractal properties.
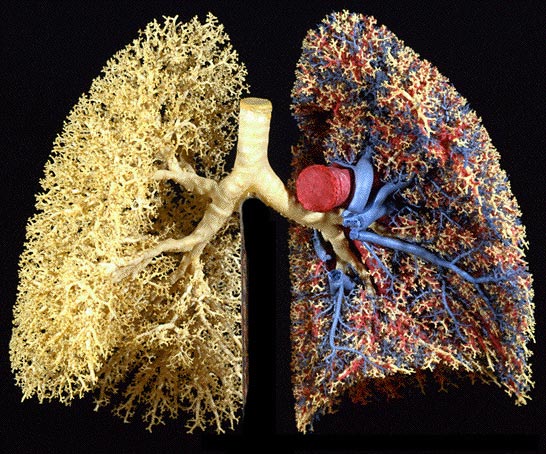
The lungs are an excellent example of a natural fractal organ. If you look at the tree upside-down (mouse over the image), you can see that the lungs share the same branching pattern as the trees.
And it is for good reason! Both the trees and lungs have evolved to serve a similar function - respiration. Since they perform a similar function, it should not be surprising that they share a similar structure. This common concept in science is known as the Structure-Function Relationship. Many of the fractals in biological systems we will explore have evolved their structures in order to perform extraordinary functions.
In the case of Lungs and Trees, they both breathe. In animals, the lungs breathe in oxygen and breathe out CO2. In plants, the process happens in reverse. Thus animals and plants are two halves of the same respiratory cycle. But here's the key to their shared structure: They both need a large surface area to function well. The amount of gas (O2 and CO2) that can be exchanged through the leaves on a tree or the
lungs in an animal is directly proportional to their total surface area. Although the volume of a pair of human lungs is only ~4 - 6 litres, the surface area of the same pair of lungs is between 50 and 100 square meters. That's about the same area as a tennis court!
Surface Area : Volume Ratio
In non-fractal shapes, from traditional geometry, there's a simple and important relationship between surface area and volume. Let's examine the surface area to volume ratio for a sphere, a simple, non-fractal shape.
The surface area for a sphere, SA = 4πR2, and the volume of a sphere, V = 4/3πR3. So the ratio of SA / V is simply 3/R, as the π's and most of the the R's cancel out. This is an inverse function, and it means that when R is large, the SA:V ratio is small, and when R is small, the SA:V ratio is large. We can plot the relationship, and see that it forms a hyperbola.
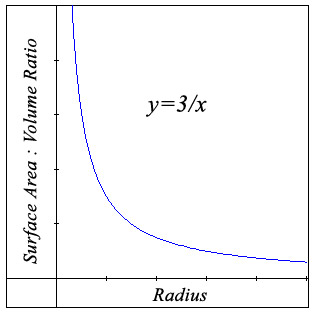 Questions: What is the SA:V ratio for our lungs? [ ] Assume the lungs have a Surface Area of 100 m2 and a Volume of 5 liters. Give your answer first in m2/L Now, to make this measurement more useful, we need to convert liters to cubic meters. There are 1000 liters in a cubic meter. Calculate the Surface Area : Volume ratio of the lungs in m2/m3 [ ] Remember the formula for SA : V for a sphere is 3/R. How small must a sphere be to have the same SA : V ratio as our lungs? R = [ ] The lesson? Fractal branching geometry provides an incredibly useful way to make a very large surface area extremely compact. To breathe, our lungs need a surface area the size of a tennis court. Imagine if we had to carry around with us big, flat, non-fractal lungs 10 meters wide and 10 meters tall! Thank goodness for fractals! |
|
<- PREVIOUS NEXT -> © Fractal Foundation. |