The Mandelbrot Set

Exploring Infinity

And now, at last, we begin our exploration of one of the most amazing objects in all of mathematics - the famed Mandelbrot Set. Let us discover the infinite beauty... of algebra!

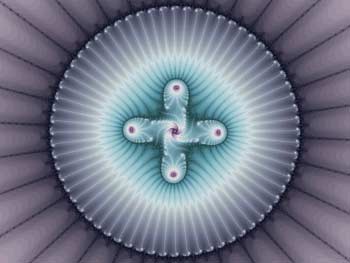
Play with the fractal above for a while. Explore the details on the edge. You can find (literally) countless examples of Self-Similar patterns within the Mandelbrot Set. But interestingly, unlike the simple IFS fractals from the previous chapter, which show the same kind of detail everywhere, at all scales, the Mandelbrot Set is NOT perfectly self-similar. The styles of the objects from different parts of this fractal are dramatically different. Furthermore, the patterns can actually GAIN in complexity and beauty the deeper you explore.

One of the most spectacular discoveries in the Mandelbrot Set is that as you zoom into it, you can find an infinite number of tiny copies of the entire object. And every one of these replicas is just as complicated as the original, and you could explore the details around the edge of a replica as well. And then of course, you can find even smaller replicas, around the replicas, and so on. <Ctrl>-click to zoom out of the replicas above to discover where in the main Mandelbrot Set they come from.

Amazingly, while there is an infinite diversity of shapes and patterns in the Mandelbrot Set, the rules that govern the way the shapes behave are universal. We will explore some of the structural rules that operate within this fractal, which will shed light on the complex behavior of simple equations. You will also be able to predict what shapes you will find in different areas, and how they connect, branch, twist and interact, allowing you to discover virtually any fractal pattern you can imagine.

Ultra-deep details from within the Mandelbrot Set. Images range from a depth of 1074 magnification to deeper than 10245 power. Images courtesy of Jonathan Leavitt, Affiniteaser Emergent.

Handling the Scale

The four images above are all inconceivably tiny details from different areas deep within the Mandelbrot Set, and are far smaller than anything in the real universe. (And they are all connected!) To manage the sizes of these mathematical objects, we must use Exponential Notation, which allows us to easily manipulate gigantic and miniscule numbers.

A reasonable estimate for the size of the universe is 100 billion light years, or roughly 10²⁷ meters. At the other end of the range, the smallest theoretical scale - known as the Planck Length^REF - is approximately 10^-35 meters. To find the difference in scale, we simply subract the exponents: 27 - (-35) = 62 orders of magnitude! This is the entire range of scale from the smallest to the largest in our universe.

To put that number in perspective,
10³ is a thousand,
10⁶ is a million,
10⁹ is a billion,
10¹² is a trillion.
10²⁴ is a trillion trillion
10³⁶ is a trillion trillion trillion
So 10⁶², the range from the smallest to the largest scales in physics, is actually much smaller than the scale of the images shown above. The range of scale between the whole Mandelbrot Set and the zoomed-in detail at the top right, which is magnified 10⁷⁴ times is 10¹², or a trillion times bigger than the whole scale of our universe. And yet these incredibly tiny images just barely scratch the surface of the infinite Mandelbrot Set.

Question:
The deepest of the four images above, on the top left, is magnified 10²⁴⁵ times.
How much larger is this fractal image then that on the top right, at 10⁷⁴? Give the exponenent: [ ]

How much larger is this deepest image then the entire universe? Give the exponenent: [ ]



Next, you will learn your way around the Mandelbrot Set...