Strange AttractorsThe Henon Attractor
The Henon attractor can be thought of as a sheet that is repeatedly folded and stretched. Imagine taking a ball of dough and rolling it out flat with a rolling pin.
Then fold it in half and roll it out flat again. Then fold it again and roll it out flat again. Repeat as long as you have patience. This foldeded fractal pattern
illustrates an important part of chaos theory, called mixing, which means that two a cluster of points in the dough that start close together will end up
thoroughly distributed throughout the dough, i.e. mixed. This describes a fractal system, because it describes an infinitely complex pattern formed by repeating a simple
process.
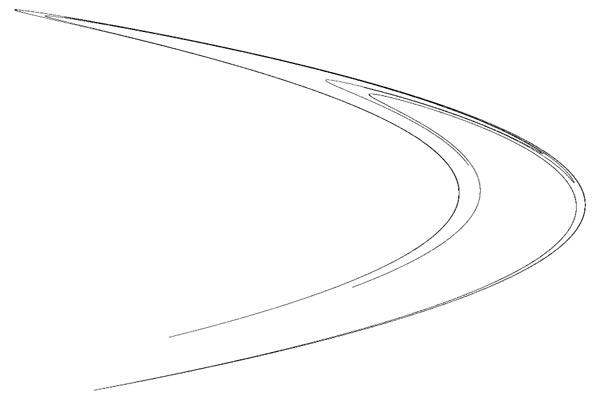 A simple view of the folded-sheet Henon Map. We can model this system mathematically with a simple set of iterated equations: Xn+1 = Yn + 1 - a Xn2 Yn+1 = b Xn By choosing different values of a and b, and iterating hundreds of times, we can see different behaviors of this complex folded sheet.
Click to zoom into the images below, and <Ctrl>-click to zoom out.
Sometimes very strange patterns emerge from the Henon Attractor, such as the spirals below: |
|
<- PREVIOUS NEXT -> © Fractal Foundation. |